

It is obtained by replacing the nonprincipal ultrafilter in fully nonstandard analysis with the more classical Fréchet filter of cofinite subsets of the natural numbers, which is the filter that implicitly underlies the concept of the classical limit of a sequence when the underlying asymptotic parameter goes off to infinity.
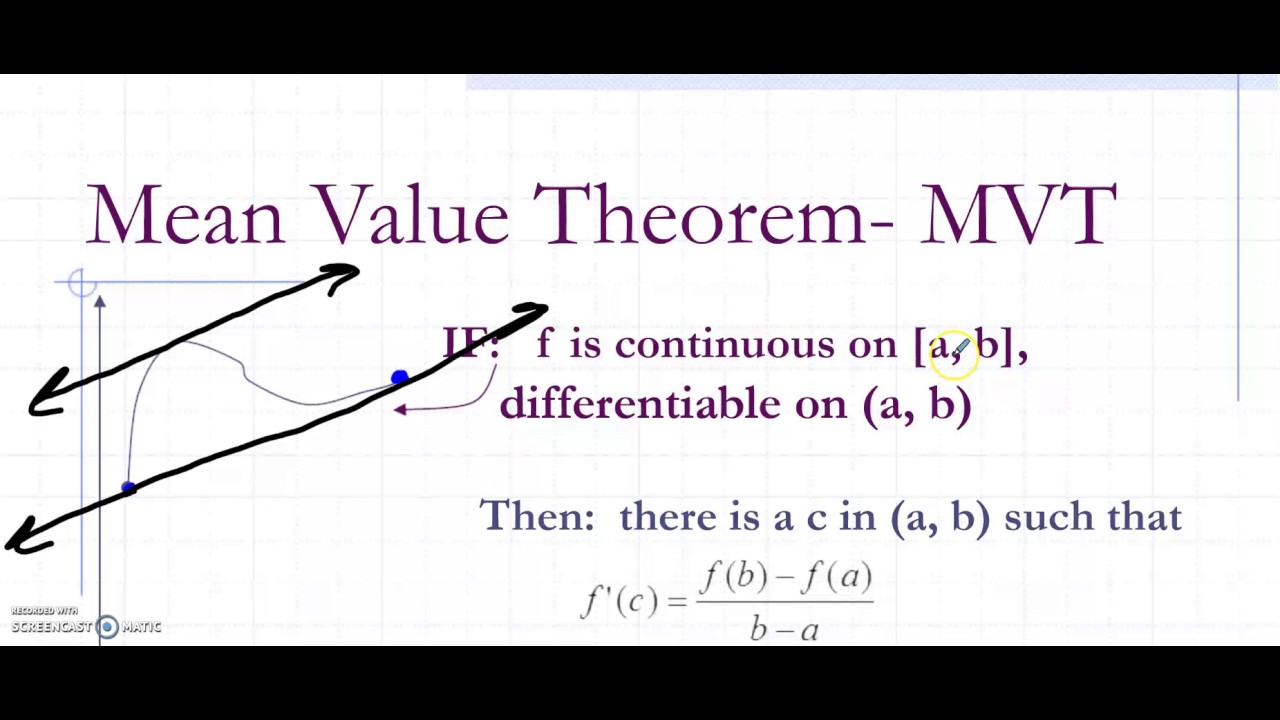
#Proof of mean value theorem using infinitesimals full#
There is however a “cheap” version of nonstandard analysis which is less powerful than the full version, but is not as infinitary in that it is constructive (in the sense of not requiring any sort of choice-type axiom), and which can be translated into standard analysis somewhat more easily than a fully nonstandard argument indeed, a cheap nonstandard argument can often be presented (by judicious use of asymptotic notation) in a way which is nearly indistinguishable from a standard one. From a more practical viewpoint, because of the presence of the infinitary ultrafilter, it can be quite difficult (though usually not impossible, with sufficient patience and effort) to take a finitary result proven via nonstandard analysis and coax an effective quantitative bound from it.

As such, ultrafilters definitely belong to the “infinitary” side of mathematics, and one may feel that it is inappropriate to use such tools for “finitary” mathematical applications, such as those which arise in hard analysis. Closely related to this is the fact that one cannot actually write down any explicit example of a nonprincipal ultrafilter, but must instead rely on nonconstructive tools such as Zorn’s lemma, the Hahn-Banach theorem, Tychonoff’s theorem, the Stone-Cech compactification, or the boolean prime ideal theorem to locate one. The most notable one is that their very existence requires the axiom of choice (or more precisely, a weaker form of this axiom known as the boolean prime ideal theorem). On the other hand, nonprincipal ultrafilters do have some unappealing features. Once one is comfortable with ultrafilters and ultrapowers, this becomes quite a simple and elegant construction, and greatly demystifies the nature of nonstandard analysis. To build a nonstandard universe from a standard one, the most common approach is to take an ultrapower of with respect to some non-principal ultrafilter over the natural numbers see e.g. The ability to rigorously manipulate infinitesimals is of course one of the most well-known advantages of working with nonstandard analysis. (See this previous blog post for more on the analogy between the use of nonstandard analysis and the use of metric completions.) Furthermore, by viewing both the standard and nonstandard universes externally (placing them both inside a larger metatheory, such as a model of Zermelo-Frankel-Choice (ZFC) set theory in some more advanced set-theoretic applications one may also wish to add some large cardinal axioms), one can place some useful additional definitions and constructions on these universes, such as defining the concept of an infinitesimal nonstandard number (a number which is smaller in magnitude than any positive standard number). (For instance, because Fermat’s last theorem is known to be true for standard natural numbers, it is automatically true for nonstandard natural numbers as well.) However, the nonstandard universe also enjoys some additional useful properties that the standard one does not, most notably the countable saturation property, which is a property somewhat analogous to the completeness property of a metric space much as metric completeness allows one to assert that the intersection of a countable family of nested closed balls is non-empty, countable saturation allows one to assert that the intersection of a countable family of nested satisfiable formulae is simultaneously satisfiable. This nonstandard universe enjoys many of the same properties as the standard one in particular, we have the transfer principle that asserts that any statement in the language of first order logic is true in the standard universe if and only if it is true in the nonstandard one. into a larger nonstandard universe of nonstandard numbers, nonstandard sets, nonstandard functions, etc., somewhat analogously to how one places the real numbers inside the complex numbers, or the rationals inside the reals. Nonstandard analysis is a mathematical framework in which one extends the standard mathematical universe of standard numbers, standard sets, standard functions, etc.


 0 kommentar(er)
0 kommentar(er)
